Conjunction Junction - Wait, what's conjunction?

The other night as we drove home late from softball, my wife started asking me a few questions about astronomy terms, and so I thought I might write down a few of them.

• Conjunction: A conjunction is when two (or rarely three or more) objects are close together in the sky.  This is typically spoken of in terms of the planets. For instance, just this week Mercury and Venus experienced conjunction, and were very close in the sky.  With so many moving objects in the sky, a conjunction of some sort occurs just about every month -- and certainly so if you include the moon as one of the objects 

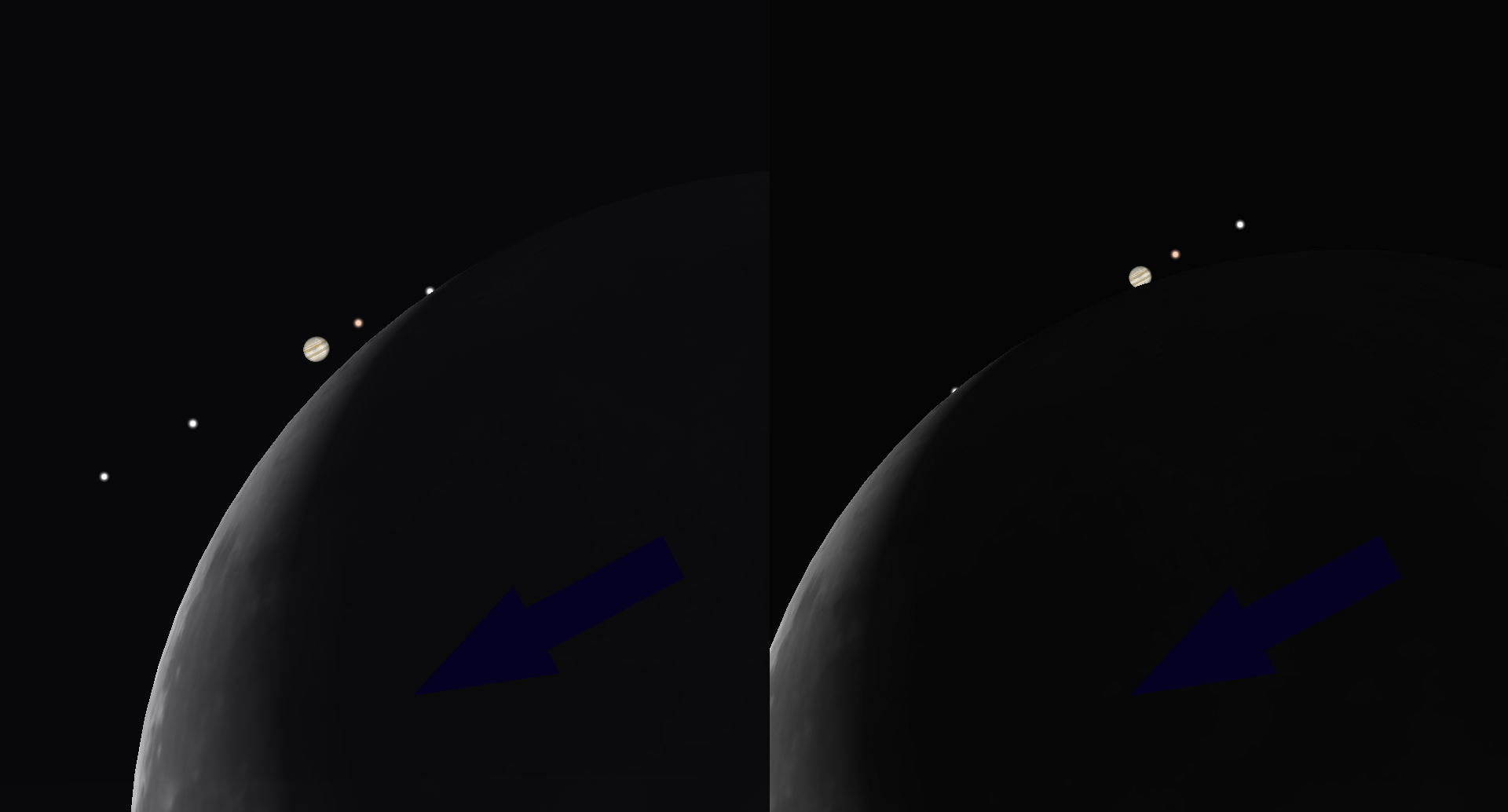Jupiter and it's moons
 "being occulted"?
(I don't think that's proper English)

• Occultation: A very special conjunction where objects appear so close together in the sky that one object actually passes behind another. The most common types are when the moon passes in front of some object, and for a short time, that object is hidden behind it. I have never had a chance to observe this. Here is a list of lunar occultations for this year and you can see that the moon passes in front of stars all the time (nearly every day) but in front of major planets only a few times. And during those times, you can only see the occultations from generally small locations on earth. 

• Transit: A transit is another type of conjunction, when a smaller object moves in front of another bigger object. The most famous transit is when Venus transits the sun, an event that occurs twice every 120 years or so. The most recent was June 5, 2012, so I'm sorry -- if you didn't see it then, you probably won't see it ever.  I made sure to watch it, and took this picture. The transits of Venus in 1639, 1761 and 1769 are of historical interest, because they helped scientists get an accurate measure of the distance from the earth to the sun.

• Syzygy: This is just too cool of a word to leave out, even though I've never seen it written anywhere except in a glossary of astronomical terms. It's a great Scrabble word, worth 25 points, for those rare (impossible actually) occasions when you have 3 y's.  Essentially, a syzygy is whenever three astronomical objects are all in a line.

• Eclipse: An eclipse is when the sun, moon, and earth are in syzygy -- and depending on the order of the three, and when the syzygy occurs, you might experience an eclipse.  Every 14.5 days the three are aligned in some way, but most of the time the moon is slightly above or below perfect alignment, and so only a handful of times each year does some kind of an eclipse occur.  Here's the next ten years of eclipses.  The next total solar eclipse that will be fully visible in the North America will be on August 21, 2017, an event I'm planning on driving down to see. 

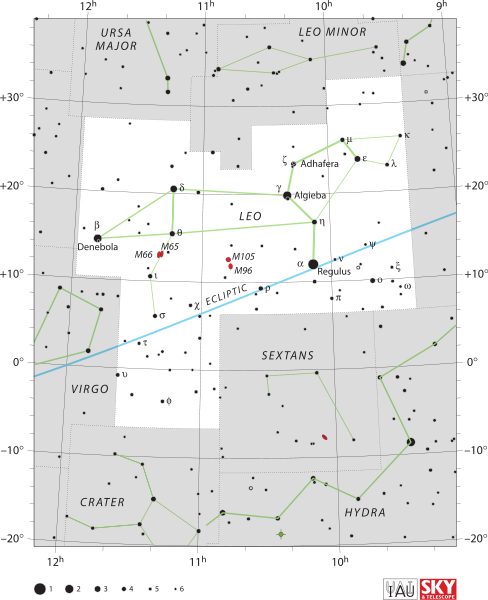
• Opposition: When a planet is at opposition, that means it is on the opposite side of the earth from sun. This is for the planets further away from the sun then earth -- and is usually the best time to observe them.  The planet is usually the brightest then -- and highest in the sky (along the local meridian) at midnight.
  
• Elongation: Elongation occurs for inferior planets (Venus and Mercury) and is when appears the farthest away from the sun. This marks the best times to observe Venus and Mercury -- when they are their brightest, and furthest away from the sun's blinding glare. 

Welcome to the dark side -- here are your cookies...

...Aw man... why are they oatmeal raisin!? Gross!

So, I haven't written a ton in the last few months, because I've been a little overwhelmed.  Most of it was just normal, end-of-the-school-year busyness (business?) of trying to finish off four different classes and grade 140 exams.

But another overwhelming thing has been switching over to using a mac.  

You see, over spring break, my household computer died -- the hard drive completely crashed. Besides losing all my data, papers from classes, pictures and videos of our kids first two years of life, etc, it also meant I didn't have a way to work from home anymore. At the time, I was spending at least 10 hours a week doing something from home (mostly in the 4:30-6:00am time frame...).  Accomplishing this all at school would have been a difficult move.

Not sure if I agree -- it was just a cool picture

Fortunately, I was given a laptop to work with. The only thing was -- it was MacBook Pro. Our school is in the initial stages of a move toward 1:1 access (every student has their own computer) and the teacher's laptops had just come in. We were given our laptops with a simple suggestion: play with them.  Get familiar with them.  

I had no choice really, but to dive in pretty quickly. Fixing or replacing my windows machine was not in our financial budget, nor did my personal time budget have room to try to make repairs. So, I began doing everything I used to do on a brand new machine.

After 10 weeks, I can finally say that I like this new machine. I still find myself behaving at times as though I'm on a PC (I keep pressing "Alt F-S" to save something and otherwise trying to access the menubar) but I am getting used to many of the changes. I won't say that I like this machine better then a PC yet -- but at least I've stopped cursing under my breath as I use it. If I end up ever liking it better, it will probably be because of QuickSilver -- but that's another post for another day. 

More posts will follow, I promise -- but it's still slow going. 

Reflections/Refractions on a rainbow:

Today we had a thunderstorm roll through an hour before sunset, and afterward a beautiful, full, double rainbow appeared.  It was so beautiful that I woke my daughter Abby up (actually, she hadn't quite fallen asleep yet) to take her outside and see it.  After about one minute Abby became more fascinated in the neighbors dog, but still, she said it was pretty.

Afterward, I came in and by the time I had posted these pictures to facebook, everyone else had already posted pictures to facebook about the same rainbow.  It reminded me of the second to last page of my difficult physics exam this semester, which was all themed about different types of severe weather, such as the energy of a falling hailstone to the current in a lightning bolt and the centripetal force of cows stuck in a tornado.  At the end of the exam, I posted this page, reflecting on the promise God made to never again destroy the world with a flood.  In it, I describe how each one of us sees our own individual rainbow - a testament to the way that God gifts each one of us separately and uniquely.

A rainbow is God's sign that he provided to us as a reminder of his covenant to never again destroy all life with a flood. Though God is the speaker in Genesis 9:16, I like to claim the verse for myself that says: "Whenever the rainbow appears in the clouds, I will see it and remember the everlasting covenant between God and all living creatures of every kind on the earth." It reminds me that though the storms of life will come, my God will not let me go.

A rainbow is a consequence of light from the sun being refracted as it enters a water droplet, reflected off the back of the drop, and refracted again as it leaves. This refraction causes the light to split into different colors. Each droplet sends a specific wavelength of light back to your eyes, which you interpret as a specific color. The entire collection of water droplets in the sky, all producing different colors -- or, if you will, all singing different notes -- produces the symphony of light that you enjoy.

Even more amazing is the fact that the person right next you is experiencing their own unique rainbow, as the rays of light necessarily must travel at different angles to reach their eyes. Therefore, a droplet that you see as red, might be producing yellow for your neighbor, and a droplet they see as violet, you might not even see at all. 

Reflect (No pun intended. Ok, maybe a little.) on these thoughts for a moment, and then proceed to the final page to share some of your own thoughts from the entire year. If time allows, feel free to additionally share some of your own thoughts on rainbows on this page.

How big a number is 400!

The other day I was teaching permutations to my Algebra 2 students, and casually asked how many different arrangements are there for our whole school body to be placed for a picture. I don't know the exact number, but I typically use 400 for estimates (yes -- I make enough estimates for our school that I have a "typical"). I knew immediately that the answer was 400! (which is 400*399*398*...*3*2*1 for those of you who have never seen the ! notation before).

My problem was that I had no concept of just how big 400! really was.  How many digits long is that number?

My initial thinking was to simply type it in the calculator, but it was too big for my TI-84 to handle.  That meant it was more than 100 digits long -- but is it more than 400 digits long? 1000 digits?  How can I answer this?

Eventually I think logarithms will be the answer, but for now let's see if we can set some upper and lower limits on things.  Since each of the digits in the multiplication from 400 down to 1 is less than 3 digits long, then the whole product must be less than 400*3 or 1200 digits long.  Since most of the digits are 2 or more digits long, it's safe to assume it must be more than 400 digits long, but just how many are there?

More formally:
   400! = 400*399*398*...*3*2*1 < 1000*1000*1000...  = 1000^400 = (10^3)^400 = 10^1200
   400! = 400*399*398*...*12*11*10! > 10*10*10*...*10*10*10! = (10^390)*10!

Now logarithms are a tool our PreCalc students will be tackling this next week, and could be used to answer this question, and it all hinges on the product - sum property of logarithms:

A factorial is simply a lot of multiplications, which would translate into a giant sum of lots of logarithms:

That can be summarized (pun intended...) as:

This gives me something that my calculator CAN handle -- since instead of actually trying to display the number, it simply gives me the magnitude of the number. This can be typed into a TI-84 by typing:

  sum(seq(log(N),N,1,400)) = 868.8 

This means the number 400! is equal to 10^868.8 and is therefore 869 digits long.

A quick check on wolfram alpha verifies this:

(I'm sure some of you were asking "Why didn't he just do that in the first place?" to which I simply respond "Because I didn't have to! God gave me a brain and problem solving skills for a reason!")

As a follow up question -- can I predict how many zeroes are at the end of that number?  In factorials, zeroes come after every multiple of 5.  (Technically, I would need multiples of 2 as well, but there are plenty of those, and relatively fewer multiples of 5).  

Up to 4! there are no zeroes:

  1, 2, 6, 24, 

Between 5! and 9! there is 1 zero:

  120, 720, 5040, 40320, 362880
Between 10! and 14! there are 2 zeroes:
  3628800, 39916800, 479001600, 6227020800, 87178291200
After that my calculator can't display them properly, but I hope you'll anticipate the pattern.

400 is the 400/5 80th multiple of 5, and so 400! is the first factorial to have 80 zeroes at the end of it.  A not-so-quick check on Wolfram Alpha's picture reveals that there are actually 33*3 or 99 zeroes.  Extras!?

That's because there are more multiples of 5 -- 25, 50, 75, ... 400 each contain 2 factors of 5, and 125, 250, 375 each contain three.  Counting these all up should reveal 99 factors of 5 (and way more factors of 2):
  80 Multiples of 5: (5, 10, 15, ..., 390, 395, 400)
  16 Multiples of 25: (25, 50, 75, ... 350, 375, 400)
  3 Multiples of 125: (125, 250, 375)
  99 total factors of 5 therefore 99 zeroes.

And for completeness (since I kept claiming there were way more factors of 2 than 5).
  200 Multiples of 2
  100 Multiples of 4
  50 Multiples of 8
  25 Multiples of 16
  12 Multiples of 32
   6 Multiples of 64
   3 Multiples of 128
   1 Multiple of 256:
  397 Total factors of 2.

What this means is that the prime factorization of 400! contains (among other things) 2^397 and 5^99.
What's the largest prime in 400! is a question for a different night. (Oh, what the heck, why not:)

What time was this photo taken?

As I read through my facebook feed, I was struck with following picture:

Immediately -- shows how much of a dork I am -- I thought "I wonder what time this picture was taken?!"

You see, as the earth rotates around the sun, shadows rotate around the objects that form them. In the northern hemisphere, these shadows rotate clockwise -- which is why clockwise is clockwise. The first clocks ever made were sundials, made by people living in the North, and then clocks were built later.

I figured I should be able to figure out the angle of the shadow of the arch and use it to figure out what time of day the picture was taken.  I could also figure out the date the picture was taken by looking at the length of the shadow. You see, everyday the angle of the sun at a given time changes. Right now, during the spring, the sun is higher in the sky every day at a specific time, which makes shadows shorter. Measure your shadow at 11:00am today and measure it again tomorrow and it will be smaller!

So I found a map of St. Louis, and used Geogebra to figure out the angle of the shadow of the sun, and the length of the shadow.  After about five minutes, I had placed a point on the map that represented where I thought the top of the shadow was, and had drawn a vector from that point to the point that represented the top of the arch. I compared that with the scale of the map, and estimated the length of the shadow to be about 1,000 ft.  After looking on wikipedia, I knew the height of the arch, and a little trig revealed the altitude of the sun to be about 32 degrees.

In a few more minutes I had estimated the angle of the the vector and converted that into a compass heading, which gives me the azimuth of the sun of approximately 111 degrees.  

I knew there is only two times a year where the sun has that exact altitude and azimuth, once in spring and again sometime in the fall -- and I took a chance that this picture was taken on spring break (reasonable enough right?). So I looked up the altitude and azimuth for the sun on the days during spring break:

Since the photo was tagged as uploaded on April 1* I started with that date, and found the following data in the table:

The first column is the time (AM), the second column is the altitude of the sun, and the third column is the azimuth of the sun.  I was disappointed that I didn't see my exact values in the table -- but I didn't expect to either, for two reasons:
  1. I didn't know if this was the correct date -- the picture might have been uploaded that day but taken several days (or even a half a year?!) earlier.
  2. There is some degree of uncertainty in my measurements. As I moved around the point where I thought the top of the shadow was, the angles varied somewhat. To be specific, they varied less than a degree more or less than my values, but that's significant enough to make my answers have to be estimates.

Let me treat each of these reasons separately.  Assuming the picture was actually taken on April 1, and my measurements were slightly off, I would estimate that the picture was taken around 8:34 am local time (I could be off by an hour if the website doesn't account for daylight-savings time, but I'm going to assume they were smart enough for that).

If I don't assume to know the date the picture was taken, and trust my measurements, I would argue that the picture wasn't actually taken on the 1st.  Looking at similar tables for other days, I get much closer altitude/azimuth combinations for a few days later:

 If I had nothing else to go on, I would estimate the date/time of the picture was April 3, 8:33am.

Perhaps the photo takers will provide the true answer in the comments below?

*There was some discrepancy between my wife and I as to when the picture was actually uploaded onto Facebook. It was posted April 5th, "tagged" April 1, but I have reason to doubt the "tagged" date. Only time will tell who wins our little "argument" -- although regardless of who wins, I will probably lose -- right guys?  I love you honey!

Taking Precautions - A Life Application from the Substitution Method

One of my favorite lessons to teach in Algebra 1 is the lesson on the substitution method of solving a system of equations. If your unfamiliar with the method, but interested, you may watch my lesson of it on youtube. As we talked about it this week, the lesson took a more spiritual turn than I had expected.

One of the first steps in the substitution method is to work to isolate one of the variables in one of the equations. Often you have some choice in which variable you'd like to isolate.  For example, in the system below, which variable would you choose to get alone?

2x + 3y = 11 and x + 5y = 16

If you chose the x in the second equation, then I would agree with you, and that's what I suggest to my students. The choice is so obvious to me, that I struggle sometimes to understand why my students don't see that. 

When addressing some students attempts at solving this with the substitution method, I approached a student who had a completely wrong answer (it's x=1 and y=3 by the way) and started looking through their work to find their mistake(s). After seeing tons of fractions and negative signs, I realized that they had made life much more difficult for themselves then they needed to. They had tried to solve the first equation for y, which produces the ugly y= -2/3x + 11/3 which then needs to be substituted into the other equation and multiplied by 5. Somewhere in the work that follows they must have made a mistake or two, but I couldn't make heads or tails of it with all the horrible fractions.

I told them that they're mistake was choosing to solve for y first, instead of finding x. They disagreed and told me "You said you can choose any of them!" which was correct, but not the full truth. I said you COULD choose any of them, but there is usually a wiser choice. 

In sharing this experience with the rest of the class, I told them how I choose the variable that doesn't have a coefficient in front of them, because I won't have to divide. Division brings fractions, and fractions is just one additional difficulty that can trip us up. I told them how in similar situations, I'll choose an equation with all positives instead of negatives to work with because those negative signs are just one more potential pitfall. 

This lead me to recite the verse from Hebrews saying "Let us throw off everything that hinders, and the sin that so easily entangles, and let us run with perseverance the race marked out for us."  I told them of how sometimes the decisions we make that seem relatively arbitrary often have important consequences, and can put us in paths that might trip us up. Over the years, I remember many times making conscious decisions one way or the other to try to avoid temptations or opportunities to fail: 

• I tie knots on the ends of my shoe and sweatshirt strings to make sure to keep them from sliding through
  
• Our family does not have cable television. Part of that is because we can't afford it, but even if we could, we decided long ago we did not want that to be a temptation because we knew that we would easily fall victim to it and watch hours of television that we don't REALLY want to. We do watch tv, but we decide what we're going to watch and either watch online or go somewhere and watch with others.
  
• In high school and college, I struggled with looking at inappropriate material online, and so many weekends when my roommates would be going away, I made them take internet cable away with them, so that I couldn't possible give in to the temptation even if I wanted to. Now web services like SafeEyes, CovenantEyes, accountability partners, and having a centralized computer instead of computers in bedrooms are strong suggestions to help provide internet safety.
  
• On a lighter note, I put my keys in the fridge on leftovers that need to come home. Or on my guitar case, so that I won't forget to bring my guitar home from school. I've even once put my keys on my daughters car seat so that I wouldn't leave her behind. (Unfortunately, I have read of parents who had left their child behind in the back seats of cars on hot sunny days because they were sleeping.)
  
• I always lock my car with my car keys button, and never lock the car with the button on the door. One time locking my keys in the car at Michigan Adventure and my wife travelling out to rescue me is enough.
  
• I now have a toothbrush and toothpaste in my lunch box. While I still haven't developed the habit I'd like (and need) I have increased the likelihood of me making that wise choice to brush after school because my stuff is always there
  
• I've removed my facebook shortcuts and apps from my phone and computer. Why I haven't just eliminated my account entirely, I don't know -- but now I have to purposefully choose to visit it instead of just going there out of habit and ending up an hour later wondering what happened. At the same time, I've made it easier to find my Bible.

What sort of things do you do to help yourself remember to do good things, or avoid falling into trouble?

Square Roots as Line Segments

Each of the square roots from √1 to √17
 are shown as the colored segments
Image by Rocky Roer
Made in Geogebra

One thing I wish my students and I had a better grasp of was square roots. We work with integer numbers well enough, but often struggle to make any sense at all of other perfectly valid numbers like square roots. It is unnatural to think of them as numbers, or lengths at all, and perhaps additional understanding could be gained from seeing them like that?

One thing I like to do is realize that each square root really is just a number. Many of them get a bad rep simply because they can't be written in fully written in decimal notation, but they can easily be drawn. Each of the square roots can be constructed quite easily, as illustrated in the picture to the right.  The first 17 square roots are drawn there, simply by drawing a unit length, constructing a perpendicular segment, drawing a unit circle, finding an intersection, and repeating. I stopped at 17 only because if I went further they would have started overlapping and I didn't like the way that looked.

Another thing I have found a little enlightening is a variation in simplest radical form. In PreCalc we've been simplifying expressions like tan(30). That process produces:

That last expression of √3/3 seems devoid of meaning, but is the required "simplified radical form" called for. I have found some enlightenment coming from describing that instead as:

This helps me to have an idea of that length -- it's just a fraction of the √3 length. Other trig segments like √3/2 and √2/2 and such could also be expressed as 1/2*√3  or 1/2*√2 and it's a step towards increasing my understanding of what those segments really are.

In our last unit, sometimes my students found values like sin(15) which is an especially ugly exact value:

Written as 1/4(√6) - 1/4(√2) is not significantly better, but helps me to realize how this number could be constructed. I could take a fraction of the yellow green segment and take away a fraction of the red segment above and make it.  Or alternately, 1/4 (√6 - √2) suggests take the yellow-green segment and chop off a red segment. Save that piece cause you'll use it many times (for secant and cosecants of 15 and 75 for instance) but take a fourth of it for now.

Now if only this understanding would help us avoid the temptation to "simplify" √6 - √2 and make it √4.

Some Geogebra Hints

Perhaps I should have made
 a real snowman instead
of playing with fake ones
Image by Benice

During our last snow day, I spent a large amount of time (for fear of embarrassment, I will not specify how long...) playing with geogebra. Along the way I learned a handful of tricks that I wanted to write down in one place. So, without further ado, here's an assorted (not random) list of tricks for working with geogebra:

• Right-click and drag draws a box and zooms in on that box
• Ctrl-Click and drag grabs the screen and moves it
• Ctrl-Alt-Delete-Shift-Right-Click and drag infects your computer with millions of geogebra viruses. Don't try it -- the rest of my snow day was spent purging my hard drive and trying to save pictures of my daughters.
• Entering a point with a semi-colon enters it in polar coordinates
    Ex: (4; 1) puts a point at a radius of 4 and angle of 1 radian (around 57degrees)
    Ex: (4; 30°) puts a point at a radius of 4 and an angle of 30° -- you can find the degree symbol off to the right if you click on the greek letter alpha and find degrees symbol
• Actually, you can insert a degree symbol while typing by pressing Alt o
• You can plot a complex number by using the imaginary number i, which you'll have to type using Alt i
• To plot a function in polar form:
1. Define your function in f(x) notation
2. Create a slider to act as the Theta settings (from 0, to 2*pi, by pi/100 is good)
3. Create a curve with the command:
       curve[f(t)*cos(t), f(t)*sin(t), t, 0, theta]
   To watch someone describe this process watch this video.
• Actually, you can insert a theta symbol by pressing Alt-t 
• and a pi symbol while typing by pressing Alt - you guessed it - p. If only microsoft word was that easy.
• To put a picture into Geogebra is fairly easy, but to describe exactly where it goes, right click on it and go to position tab. Then you can type in the coordinates of the corners of the picture directly, or you can attach these coordinates to sliders so that you can control them dynamically. Often I like to put the picture as a background object, so that other things lie on top of it.

Are you smarter than a calculator?

Lately, I have been noticing how dumb our calculators are. It's become kind of a running theme in my classes, where I've been teaching how to use different graphing tools, and I say several times a week "and remember, you have to be smarter than your calculator" or "you have to help your calculator..."

The calculator doesn't think like humans. God created us--not calculators--in his image, and I believe one aspect of that is the ability we have to reason, to notice patterns, to create, to organize, to see. These are all things that calculators, and in general computers or machines, are all pretty bad at. They are improving, because our minds are helping to generate better and better machinery, but they still don't work like humans.

Perhaps the best example of this is the Captcha messages at the bottom of so many websites. Computers and bots are still horrible at "seeing" things. Most humans can interpret those pictures and type letters or numbers properly, but that relatively simple operation is difficult for most computers. That's because we think about the problems entirely differently.

Likewise, our calculators think about calculations entirely differently than us. We can think algebraically and manipulate symbols, variables, and even numbers in symbolic ways that allows us to simplify problems, or calculate values exactly. Most calculators don't think in that way at all, but are programmed with different algorithms that work with really precise approximations and quick calculations. Even the slowest earliest calculators can do this sort of thing faster than all but the freakest of humans -- but I haven't seen any calculators that are good at playing What's the Word.

Here's a bullet list of items that I've noticed lately:

• In PreCalc we've been studying complex numbers. One assignment the other day was to calculate i¹⁷ which is easy to calculate by recognizing a pattern.  i, i⁵, i⁹, i¹³, and i¹⁷ are all equal to the purely imaginary number i, but the calculator spit out -1E-13 + i.  Yes, the -1E-13 is a ridiculously small number, close to zero, but it shouldn't be there AT ALL! What strange algorithm does the calculator use to calculate that instead of just recognizing the pattern like humans?
• Similarly, some versions of the calculator were not able to convert some of our operations involving complex numbers into exact fraction form -- where as we could. Some calculated approximations (admittedly better approximations than we could find in anything short of five/ten minutes) but several others gave an ERR: data type message instead
• In Algebra 2, we gave been using the calculator to calculate summations, and the notation for summations is sum(seq(function,VAR,start,end)) and we have been laughing at the fact that even though our functions only have one letter in them, we still need to write that variable again. I understand you could certainly have many variables in a function and then you'd have to specify which one is the index -- but when there's only one, you'd think the calculator would be able to figure that out.
• In Algebra 1 we've been graphing systems of equations, and numerous stupid calculator quirks have popped up. Though we set the word problems up with sensible variables like N for the number of nickels and D for the number of dimes, when we went to graph things, we had to use the letters X and Y. Again, you could maybe give your calculator the benefit of the doubt because maybe those letters are going to be used for constants (like I do in physics storing 6.67E-11 in for G) but...
• Then we try to calculate the intersection of two lines and we have to tell it which lines we're interested in and help guide it towards the solution. Seriously?! There's only two lines on the screen! And they're lines! Not curves!  
• If the intersection isn't on the visible window screen, the calculator won't be able to find it for you -- you need to realize that those lines will intersect above, left, right, etc. of the screen and adjust the window yourselves.
• And what's with providing the answer as 1.999946 when it's clearly and exactly 2?  The algorithm that calculates the intersection necessarily has limits to its precision, and sometimes those fall short. My students better not ever report an answer of x=1.999946 to me.
• To be fair, let's pick on non-TI84 calculators -- one of my newfound favorites is the app MyScript Calculator which interprets my handwriting and calculates things for me.  I'll admit, I played with it for a good 30 minutes after downloading it -- only true math nerds play with their calculators right?  But I noticed pretty quickly that it's trig values didn't always calculate properly, which was a bug that their updated version supposedly has fixed. I knew that because I knew the limits of sine and cosine values, and even had several memorized -- and also can estimate relatively well and had ideas of what the answers should be ahead of time.

The Constellation Leo

This post is the one of a series on constellations and posted throughout the year as each constellation comes into prominence.

Leo is one of my favorite constellations. It was one of the first after I fell in love with astronomy in 2008. It was the first one that I had never noticed before, but set out to find and add to my repertoire.

Leo is a lion, and one of the constellations that I feel actually looks like its supposed to:

Leo the Lion
Image by Backyard Stargazing

It reminds me of the sphinx:

What helped me to identify Leo was to find the sickle -- the curve of six or seven stars which I call the "backwards question mark".  The dot of this question mark is the brighest star in Leo, called Regulus, or Reggie for short. At #15, he is one of the brightest stars in the northern hemisphere. He lies almost exactly on the eclipitic.

Lying along the ecliptic, Leo is therefore a zodiacal constellation. This means the sun, moon, and the planets periodically pass through Leo. When I was first learning about it, in 2008, Saturn was moving around under Leo, though now it has moved on and is located in the relatively blank section of sky in Virgo and Lyra.

The sun passes through Leo from mid-August to mid-September, which makes Leo a nice constellation to look for in late winter and spring. I find it by locating the Big Dipper, and pretending it is dripping things. If it drips things down thru the cup, those drops would fall on Leo's head:

The Big Dripper and Leo

Image by Catawba Astronomy Club

Below is a more specific map of Leo.  With a telescope, Leo houses a few good Messier objects worth looking for, but none are good sights for binoculars.

Leo
Image from Wikipedia

Polar Rose Explorer

Here is a geogebra activity I made which explores polar graphs for precalc.  Just in time for Valentines: Men, be sure to make your wives and girly-friends a dozen polar roses!

If the above embedded file does not work -- you probably need to install or update Java. You can also find it on Geogebratube.

This is the Day

Today I had several hours on my own with the girls while Carrie ran errands, and as much as I love my girls -- I often dread these times.  Usually it's because I feel inadequate, especially with my littlest. I am not good at deciphering what she wants -- what's the difference between AAAHHH! and WAHHHH!?  Even if I can figure out what she wants -- I'm not as good at everything as Carrie, nor as quick at getting a bottle ready, and Ellie get's frustrated waiting for me.  

On top of my inadequacy, I also struggle with patience issues -- and lose my patience most often with my own children.

But enough about me -- I really just wanted to share a few thoughts I had while playing with Ellie. 

Today I sat her up, and put a toy in front of her so she could practice sitting up.  The toy was a little ball-like face that giggled whenever it shook. Ellie loved it, and giggled along with it for at least fifteen minutes. As I sat there enjoying it, I thought "Toy: this is your day. Until now, Ellie hasn't been strong enough to sit up and really enjoy you, and tomorrow she'll probably have grown up so far that you'll be boring. This is your day to  shine -- so giggle on little one.  Giggle on."  

I know -- really deep right?  But it did remind me of the popular Sunday school song:

This is the day
That the Lord has made
We will rejoice and be glad in it

It reminded me that our time is short. Our time as parents is short -- our kids are only young for so long!  Every time I ignore Ellie is precious time I can't get back. Will I miss my "day" someday? 

It also reminded me that our time on earth is short. We were made to honor and glorify God and how often I waste that time. Why? This is the day that the Lord has made! Giggle on!

Graphs of Inequalities

I just finished a unit on inequalities with my Algebra 1 students, and I remember in years past struggling to find a way to include number line graphs in my quizzes, notes, and slide shows.

 Over the years I've amassed a large number of these number line graphs, of different types, and thought I'd throw them up on the blog for the one or two other math teachers who might stumble across this and want them. They are in a PowerPoint format, because I found that working with shapes and lines in PowerPoint was easier than any other program I had.  If you're interested, here's a file containing them.

They are just a series of shape objects, which I alter by adjusting their size or orientation.  Once I have the graph I want, then I select all the objects, copy them, and paste them as a picture, which I can use in PowerPoint or Word.  

One thing I am still looking for is a good source of creating two dimensional graphs. If you know of something, post it in the comments below.

Record Breaking Game of Lightning

As I write this, people are supposed to be gathering at Cornerstone University, where I just finished my masters degree this past April and across the street from my school, to hopefully break the world record for "largest game of Knockout" ever.  They have a goal of having over 500 people come and play Knockout - or as I grew up calling it, Lightning.

All the way home this evening I was wondering if I was going to regret the opportunity to be there and to make history.  So I consoled myself by trying to figure out How long it would take to finish the game?

For those of you unfamiliar with the game, read the rules, and then come back.

One problem with this question is that there isn't a definite answer to it -- at least not until tomorrow when I read about it and find out how long it took.  The game potentially could go on forever, or could be over in ... well -- maybe we could start there?

Actually, let's begin with just a few estimates. I'm going to assume that on average it takes t=7 seconds for a person to get the ball, shoot an initial free throw, catch the rebound, shoot a layup, etc. and pass the ball to the next player.  Some will certainly take less time, and others more, but I need to use something.

I'll also estimate n=500 people showing up.

To begin, let's see how long it would take just to get through the first round -- that is for everyone to have touched the ball and had one attempt to knockout the player in front of them:

It will take one hour just to get everyone a chance to shoot the ball -- wow.  Already I feel better heading home!

Just for kicks, how big would the line be?  Assuming about 3 feet of space between players, it would be:

The line would be nearly a half mile long, or would likely weave back and forth nearly 10 times on the basketball courts. Of course, cornerstone's gymnasium is huge and has a jogging track around it too, so they've probably thought ahead about how the line will operate- I hope.

So how about an estimate for the minimum amount of time it would take to finish? The very least number of shots required would be if EVERY time two people came up to shoot, the person behind beat the person ahead of them. That produces a pattern of people:
  (loses)(wins)(loses)(wins)....
  This would eliminate a player every time -- so after 500 pairs, there would be one person left over.  This yields 1000 shots, or approximately 2 hours.  I wasn't willing to devote that much time to the activity -- (and yes -- I would have ended up being that last shooter because I am that good).

Of course, it's certainly going to take more than 1000 shots, because there will be many times when the back player doesn't win.  Suppose the back player wins 50% of the time?  Let me try to encode what I'm envisioning:
   (survives)(loses)(wins)(survives)(loses)(wins)
 This should require 1500 shots, or roughly 3 hours to complete.

If players are equally matched, the back player shouldn't win even that often though, because they have a little disadvantage because the player ahead has a head start.  Suppose they have a 33% chance of winning? It might look like:
  (survives)(survives)(loses)(wins)(survives)(survives)(loses)(wins)...  It takes four shots to produce a knockout now, so approximately 2000 shots or 4 hours to complete.

Several years ago, when I was in undergrad, four hours on a Friday night would probably be fun to spend on something like this massive basketball game -- but not so much anymore.

I have to admit, I'm a little disappointed in how this post turned out -- I was making things super complicated in the car thinking about probability and how many rolls it takes to roll a six and how many shots it takes for a 50% shooter to make one and making this problem way more complicated than it needed to be.  So I was anticipating pulling out all sorts of statistics and the binomial theorem, but oh well. Perhaps another day.

Greek Letter Shortcuts in Microsoft Word

When typing up notes, worksheets, and assignments, I often find myself needing to type some special mathematical symbols, such as π. Other Greek letters are useful too, such as Δ for change in. The lazy thing to do is just type pi, or delta, but there are a handful of ways to insert these symbols into a document.

Character Map

• In Windows, you can find the old Character Map -- a program I've seen since I used Windows 3.1 growing up. You can find it by pressing the Windows button and searching for character map -- and then the window on the right will pop up. In it you can find a wealth of characters that you can copy onto the clipboard and paste into whatever program you're typing in. I used it to type the π and Δ symbols above.  Though this technique can work, and work for multiple programs, I don't use it often
  
  
• In Microsoft Word and PowerPoint, you can find Insert Symbol in the ribbon along the top. Like Character Map, it gives you a list of hundreds of different symbols that you can search through to use. The window looks like this:

• If you notice the bottom of the box there is a shortcut key programmed in for the letter π. In Microsoft Word, you can use this code to enter in a π symbol without using this window -- much faster!  To use the code, type 03c0 (those are zeroes) and then hold Alt and press X.  You'll see that the 03c0 becomes a π symbol! Magic! And many other characters have a character code similar to π. My problem is that there is no way that I'll remember each of those strange character codes every two weeks or so that I need them. So I would have to look them up online and in the time it takes to do that, I could have just found it in the insert symbol box.
  
• A related technique is to use an Alt code.  Hold Alt and press 227 on number pad, then let go of Alt and you'll see a π symbol appear.  Many other letters have a Alt code, but again -- how am I supposed to remember which codes are which?  And why in the world is the Alt code for π 227 and not something reasonable like 314?!
  
• My favorite solution to this in Microsoft Word is to use the shortcut button to create my own keyboard shortcuts for each of the symbols I use often!  If you click on the button "Shortcut Key" it will bring up the following form:
  As you can see, I have created a keyboard shortcut that I'll remember, and stored it in Word so that when I return a few weeks later, I can find the π symbol more quickly!  For me, it made sense to type Alt G, P.  My line of reasoning is that it's a greek letter, Pi.  I have gone ahead and assigned shortcuts similar to this for all the Greek letters that I use on a regular basis:
• Alt G, D for lowercase delta
• Alt G, Shift-D for uppercase delta (for "Change in")
• Alt G, A for alpha (for angles)
• Alt G, B for beta (for angles)
• Alt G, Q for Theta (for angles)
• Alt G, T for Tau (torque in Physics)
• Alt G, r for Rho (density in Physics)
• Alt G, W for Omega (rotational velocity in Physics)
• Alt G Shift-W for captial Omega (Ohm symbol for resistance in Physics)
• Alt M, . for the mathematical symbol for multiplication
• Alt M, 2 for the squared symbol
• Alt M, 3 for the cubed symbol
• Alt M, R for the square root symbol 
• While on the topic, I should also mention auto-correct as an option. I remember using that quite frequently during my Spanish classes.  I made it so typing n~ and a' and such created the accents and symbols -- though if I used those characters much now I would probably create a series of Alt codes for them, perhaps with Alt S, N and Alt S, A, etc. 

Reflections on J-Term 2013

We just finished J-Term and I have so much to write about, but so little time to write, so let me just do quick summaries and if I have any time (this summer?) I'll come back and elaborate.

For those of you who don't know, at our school J-Term is a week-long opportunity for our students to take some unique classes and learn things their teachers don't normally get to do during the school year. For instance, many students took iPad video making, or an interesting Hunger Games exploration, knitting, chess class, etc. The teachers suggest offerings and the students sign up for three different classes they'd like to take.

This year I offered two classes: Astronomy (which I have taught before) and a new class which I called "Did You Get My Email?" but might more formally be called Digital Communications.

Star and Planet Locator
by Edmund Scientific

In Astronomy we learned a 15-20 constellations, discussed how to use a Planisphere, the idea of altitude and azimuth, how to find the planets along the ecliptic, and how the sun moves through different constellations (the zodiac) throughout the year. Next on the list would have been declination and right ascension, but we ran out of time.

The Star and Planet Locator made by Edmund Scientific is an great tool for teaching these concepts -- and at only $3.95 per unit it's one of the cheapest I could find.  I bought mine a few years ago and kind of remember a 25 for $50 deal so if you're interested in a classroom set, look around.

These worksheets I offered:

• Learning the Constellations: Identify the constellations you see in different maps
• Using a Star Finder:
• Using a Star Finder Part 2:
• Seasons: Questions regarding seasons and sun on star finder
• Angles Between Stars: measuring altitude and azimuth with hands, degrees, etc.
• Estimating Location of Stars: practicing altitude and azimuth with a star finder
• Celestial Coordinates: practice with declination and right ascension
• Stars and Heavens in Scripture: Bible passages referring to the stars and heavens
• The Star Clock: Using the big dipper to tell what time it is

The other class I taught was new to me -- Digital Communications. I'll admit I'm not proud of how this class turned out because I didn't put the time into it over Christmas break that I should have. In this class we learned about a ton of different technologies, leaning quite heavily on "How Stuff Works" descriptions of: the telegram, telephone, television, computers, hard drive, cd player, text messaging, email, radio, etc. We also studied binary numbers, and spent some time describing how computers convert all information into numbers, which are all converted into binary, which can mean everything can be stored ultimately as a handful of 1's and 0's somewhere.

I also did some hands on materials, though I had ambitions of doing way more. We played around with simple circuits, hooking up batteries and lights. We made a few electromagnets, and I showed them a homemade "byte" -- 8 bits -- which I made with just a piece of wood, 8 nails, and a about 40 ft of wire. I never used it in anyway besides holding it up occasionally when we discussed that 8-bits define a character in Ascii, or that three of these 8-bits define a color of an individual pixel in a picture, and so on.

I learned from this that I enjoy doing things hands-on and should take more time to make that happen in my classroom. I learned that radio shack has a lot of small circuit components for sale, such as LED's, solar panels, resistors, switches, etc and I have a lot of material now that I'll be able to use in our electricity unit in physics.  And I learned that classes will survive, even if you are fully prepped for them. Maybe that wasn't the lesson I should have learned -- but I did.